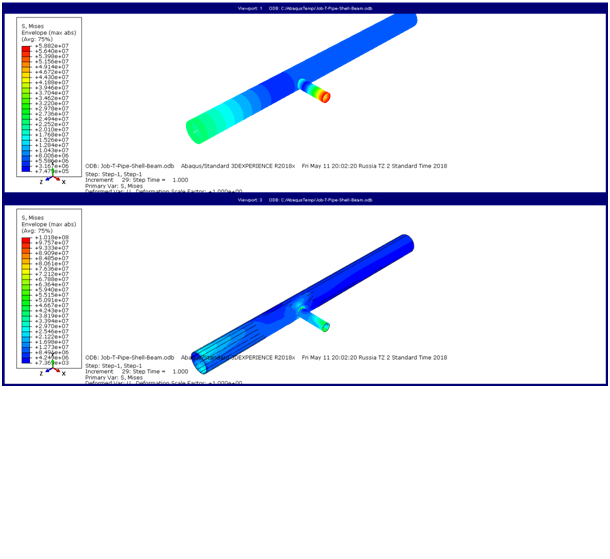
Stress simulations for pipelines
Checking the pipeline designs, I use shell finite-element theory for stress simulations.
In almost every project, I find significant differences in stresses and strains between my stress simulations and stress simulations that were previously performed by somebody with the application of beam finite element theory.
For example - an error in Mises's stress generally exceeds 50% in places where the pipelines are supported by supports.
Therefore, I often have to prove the reliability of my stress simulations to the designers - which it is often quite difficult and it takes a lot of time.
I would be glad to hear the opinion of colleagues (stress engineers).


As an adult, she is passionate about creating new ideas that help others achieve happiness and personal fulfillment, and these wonderful books are part of realizing that goal. The Makings Of Us
We are top achievers, and now we have taken responsibility of your projects. We offer writing service at an affordable price.
E book Writing Service UK
Nice work! Required one correction to get the shading completely great. I was unable to be more joyful. I would work with this craftsman once more.
Fresh Logo Design
Our all Academic writers follow a logical structure, straightforward structure. Our In Our academic service, we provide introduction background information, lays out the scope and direction of the essay, and states he thesis.
In our papers the body paragraphs support the thesis statement, with each body paragraph elaborating on one supporting point.
In our papers the conclusion refers back to the thesis, summarizes the main points, and highlights the implications of the paper’s finding.
Our each sentences and paragraph logically connects to the next in order to present clear arguments.
Help Hogwarts Papers
The application of the theory of girder finite elements to the stress simulations of pipelines is "illegal".
The hypothesis of FLAT sections and the Saint-Venant principle are valid only for long rods of a LIMITED profile, i.e. having transverse dimensions of ONE order!
For thin-walled beams, when one transverse dimension is much larger than the other, estimates of the relative orders of magnitude of the normal and tangential stresses cease to be valid, the hypothesis of flat sections loses force and the Saint Venant principle becomes unacceptable.
Pipe is not a beam element
Pipe is a thin cylindrical shell
Therefore, for almost all cases - the pipeline should be considered as a shell construction
In the process of loading the pipeline, the cross section ceases to be perfectly round - the curvature of the pipeline changes, both radially and in the longitudinal direction - this can not be taken into account by the theory of beam finite elements.
The beam theory of finite elements excludes the possibility of REAL simulation of the contact of pipelines with supports.
And since in the process of loading the area of contacts and their location change - the beam theory of finite elements can not give REAL values of stresses that arise in contacts.
You are mistaken in saying that the pipelines have small deformations.
Almost always - pipelines have large deformations and almost always pipelines have geometric nonlinearity.
Applying the theory of beam finite elements it is impossible to obtain distribution of stress at the intersection / conjugation of pipes, as well as at the intersection / junction of pipes with reservoirs.
Applying the theory of beam finite elements it is impossible to correctly define the boundary conditions that simulate the supports - since the boundary conditions are set to points located on the axis of symmetry of the pipeline.
In fact, the support of the pipeline to the supports occurs along the outer surface of the pipeline - thus the "error" in setting the boundary conditions will be 0.5 the radius of the pipeline.
The consequence of this is an incorrect calculation of the forces , bending and twisting moments => incorrect distribution of strain/stress in pipe.